 At Wal-Mart, CDs cost $0.50 per disk, and pens cost $1.00 each.
At Wal-Mart, CDs cost $0.50 per disk, and pens cost $1.00 each.
Which inequality best describes the number of CDs, c, that can be purchased for $5.00 or less if you have spent a part of the money on v pens?
 At Wal-Mart, CDs cost $0.50 per disk, and pens cost $1.00 each.
At Wal-Mart, CDs cost $0.50 per disk, and pens cost $1.00 each.
Which inequality best describes the number of CDs, c, that can be purchased for $5.00 or less if you have spent a part of the money on v pens?
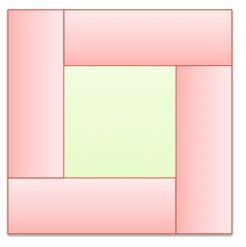
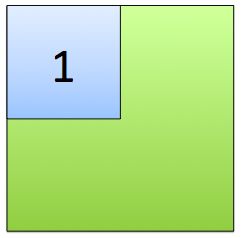 A father wishes to divide a square piece of land among his five sons.
A father wishes to divide a square piece of land among his five sons.
He gives one quarter of the land to the first son, as shown.
He divides the remaining land into four plots of equal size and shape.
Find the plot.
Source: Fixx, James F Solve It!, 1978
 John's dad pays him $8 for each correct answer he gives in his math homework and fines him $2 for each incorrect answer.
John's dad pays him $8 for each correct answer he gives in his math homework and fines him $2 for each incorrect answer.
Today, John received nothing after doing 50 problems.
How many problems did John answer correctly?
 Bob is looking for a new job. He has three offers with the same starting salary.
Bob is looking for a new job. He has three offers with the same starting salary.
Bob wants to be better paid in the first year.
Please help him to make a decision about his monthly salary raise.
 A restaurant sells a 30 cm (in diameter) pizza for $9, while their large 40 cm pizza is specially priced at $12.
A restaurant sells a 30 cm (in diameter) pizza for $9, while their large 40 cm pizza is specially priced at $12.
What is the percent discount the restaurant is offering for the large pizza?
 A real estate agent receives a 5% commission on the selling price of a house from the buyer.
A real estate agent receives a 5% commission on the selling price of a house from the buyer.
You can borrow 80% of the house price from your bank.
If you have $250 000, what is the most expensive house that you can afford?
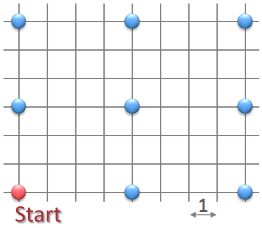 The traveling salesman problem: a salesman has to visit eight towns and return home.
The traveling salesman problem: a salesman has to visit eight towns and return home.
What is the shortest available route between the towns?
 Blind people use a special method to read texts.
Blind people use a special method to read texts.
The method was invented by the Frenchman Louis Braille in 1829.
Each symbol is raised on a base of six positions, two across in three lines.
The picture shows six first letters of the Braille alphabet.
How many different symbols can you produce on the base of six positions?
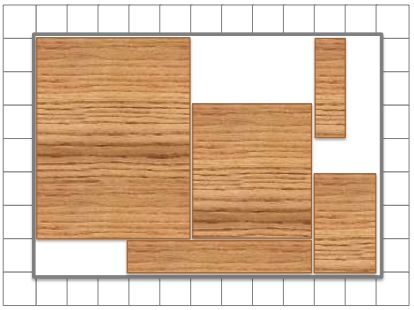 I use a room that is 7 x 11 to arrange five boxes.
I use a room that is 7 x 11 to arrange five boxes.
I would like to move the boxes to a smaller room.
What is the smallest possible area of the new rectangular room?
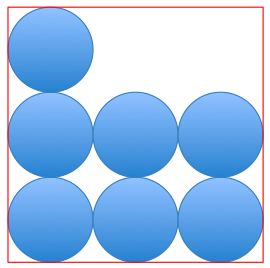 I placed seven balls into a square box.
I placed seven balls into a square box.
Is it possible to rearrange the balls in order to use a smaller square box?
If yes, how much does the box side length decrease?
 Brad is framing a small flower box by four rectangular bricks.
Brad is framing a small flower box by four rectangular bricks.
Each of the rectangles has a perimeter of 80 cm.
What is the area of the flower box including the bricks?
 Yesterday, I caught 30 fish of a certain size in a pond.
Yesterday, I caught 30 fish of a certain size in a pond.
I marked and released them without any harm.
Today I also caught and released 80 fish of the same size and noticed that there were 6 marked fish in the second catch.
How many fish of the same size are there in the pond?
The photograph courtesy of Roland Sauter
 In a casino on a Sunday, 90% of the visitors lost $100 each,
In a casino on a Sunday, 90% of the visitors lost $100 each,
9% of the visitors lost $1,000 each, and
the rest won $10,000 each.
If the profit of the casino is $80,000, how many people visited the casino?
 Several plumbers work on a pipeline installation, which 1 plumber is able to complete in 120 days.
Several plumbers work on a pipeline installation, which 1 plumber is able to complete in 120 days.
If 2 plumbers are sick, then the others work for 10 days more.
How many plumbers are in the team?
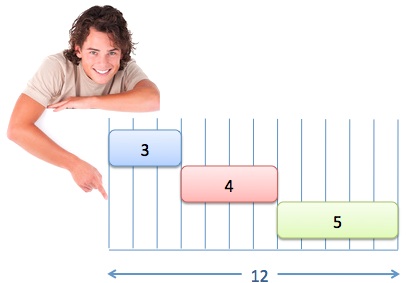 John works on a 12-month project.
John works on a 12-month project.
Forty percent of the first 3-month activity is completed.
Seventy percent of the second 4-month activity is completed.
The third 5-month activity has not been started.
What part of the project is completed?
 A project manager needs a car 3 days per week.
A project manager needs a car 3 days per week.
The daily cost of car renting is $200.
To purchase the car, it costs $26,000, and the daily car expenses are $50.
What should the project manager do if the project lasts 52 weeks?
The car is used in a toxic environment. If it is used for 6 months it is economically salvageable. If it is used for the whole year it is economically infeasible to clean it, so the vehicle is effectively worthless.
 The average hourly wages of men and women in company A are $21 and $16, respectively.
The average hourly wages of men and women in company A are $21 and $16, respectively.
The average hourly wages of men and women in company B are $20 and $15, respectively.
Twenty percent of company A employees are men.
Forty percent of company B employees are men.
Which company pays a higher average wage to their employees?
 If 9 workers can build 9 houses in 9 months,
If 9 workers can build 9 houses in 9 months,
then how many months would it take 9 workers to build 11 houses?
 Jim sells 60 percent of pineapples he had and throws away 10 percent of the remainder. On the next day, he sells two-thirds of the remainder and throws away the rest.
Jim sells 60 percent of pineapples he had and throws away 10 percent of the remainder. On the next day, he sells two-thirds of the remainder and throws away the rest.
What percent of his pineapples does Jim discard?
 A space rocket has a critical fuel system, protected by 4 independent safety components. If all 4 safety components fail, the rocket will explode after lift off. The probability that any one safety component will fail is 1/10.
A space rocket has a critical fuel system, protected by 4 independent safety components. If all 4 safety components fail, the rocket will explode after lift off. The probability that any one safety component will fail is 1/10.
If the rocket has lifted off, what is the probability that it will not crash?
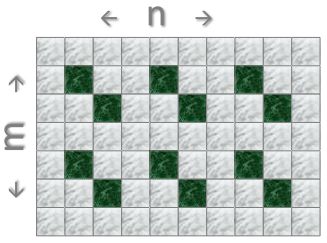 A city hall floor is to be tiled in the following pattern.
A city hall floor is to be tiled in the following pattern.
The hall measures 91 tiles x 61 tiles.
How many green tiles do we need?
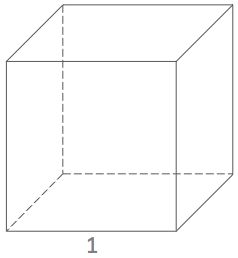 It costs $24 to paint a cube.
It costs $24 to paint a cube.
Before painting, it was cut in four pieces by two planes.
What is maximum possible cost to paint these four pieces?
 A full box with 70 matches weighs the same as four boxes with 10 matches each.
A full box with 70 matches weighs the same as four boxes with 10 matches each.
How many empty boxes weigh the same as a full box?
 If 5% of the people get 95% of the total salary paid in a county, and the average salary is $50,000, what is average salary of the highest paid people?
If 5% of the people get 95% of the total salary paid in a county, and the average salary is $50,000, what is average salary of the highest paid people?
 Anna can complete a project in 40 days.
Anna can complete a project in 40 days.
Bill can complete the project in 50 days.
Cindy can complete the project in 60 days.
Daniel can complete the project in 120 days.
Their daily wages are proportional to their performance.
Find two people who together complete the project in exactly 40 days.
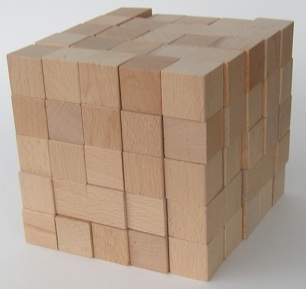 I want to construct a solid brick from wooden cubes.
I want to construct a solid brick from wooden cubes.
Its side ratio is 1 : 2 : 3.
I have 200 wooden cubes.
What is the maximum number of cubes I will use?
 There are 8 people in a village.
There are 8 people in a village.
They consume 8 coconuts altogether.
Each man eats two coconuts, each woman eats a half, and each child eats a quarter.
How many men are there?
 Inspired by Morozkin's problem :
Inspired by Morozkin's problem :
Two children started at 6:00 and each walked at a constant (different) speed.
A boy went from A to B and a girl from B to A. They met at noon and continued with no stops. The boy arrived at B at 4 p.m.
At what time did the girl arrive at A?
 Anna and Bill roll a six-sided die.
Anna and Bill roll a six-sided die.
The first person to roll a six wins.
Anna goes first.
What is the probability that she will win the game?
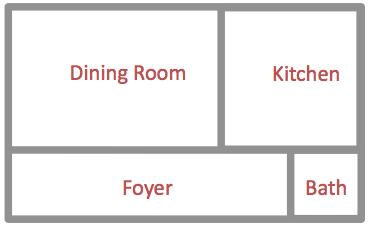 The picture shows the rectangular floor plan of the first level of a house.
The picture shows the rectangular floor plan of the first level of a house.
Both the bathroom and the kitchen are square with areas of 4 square meters and 64 square meters, respectively.
The dining room is rectangular with an area of 96 square meters.
What is the area of all 4 rooms?
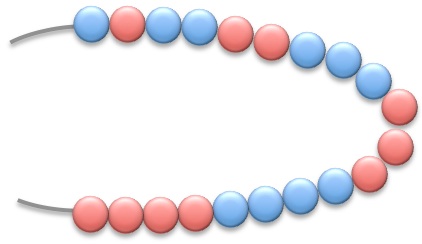 I am placing round beads: 1 blue and 1 red; then 2 blue and 2 red; then 3 blue and 3 red; and so on.
I am placing round beads: 1 blue and 1 red; then 2 blue and 2 red; then 3 blue and 3 red; and so on.
If there are 95 beads in total, how many of them are blue?
 The rear tires of my car wear out after 60,000 miles, whilst the front tires wear out twice as fast.
The rear tires of my car wear out after 60,000 miles, whilst the front tires wear out twice as fast.
Estimate how many miles I can drive if I change the tires (front and rear) at the best moment.
 80% of the women in company A are under 25 years old, 40% of the men in the company are under 25 years old, and 50% of all employees are under 25 years old.
80% of the women in company A are under 25 years old, 40% of the men in the company are under 25 years old, and 50% of all employees are under 25 years old.
What percentage of the company are men?
 If Mark had driven at 6 miles per hour (mph) faster than the state speed limit on a trip, it would have taken him 6 minutes less.
If Mark had driven at 6 miles per hour (mph) faster than the state speed limit on a trip, it would have taken him 6 minutes less.
When does he arrive if he starts at 7:00 and the state speed limit is 70 mph?
 Since the day he was born, Bill's parents have put $1000 in his bank account every year.
Since the day he was born, Bill's parents have put $1000 in his bank account every year.
Each year the account earns 1% simple interest.
How much money will he get after 20 years?
 In selling stock, John made a profit of 19% of the amount originally paid for the stock.
In selling stock, John made a profit of 19% of the amount originally paid for the stock.
He pays $99 for the transaction.
If the cost of the stock was originally $25,000 what was his final profit?
 The size of an NBA basketball court is about 29 by 16 meters.
The size of an NBA basketball court is about 29 by 16 meters.
How many courts can be planned in an 80 x 54 meter school yard, given that at least 3 meters must separate the courts?
 The population density of the Italian capital Rome is about 2,000 people per square kilometer.
The population density of the Italian capital Rome is about 2,000 people per square kilometer.
How many square meters does a citizen of the city get?
There are 1000 meters in one kilometer.
Don't count tourists.
 Each week, Mrs. Smith gives her students as many worksheets as their age.
Each week, Mrs. Smith gives her students as many worksheets as their age.
For example, Jane, who is 7-years old, gets 7 worksheets for this week.
If there are 20 students aged 7 - 10 years, how many worksheets should Mrs. Smith prepare for this week?
 Jane pedaled downhill at 20 km/h (kilometers per hour) and it took 2 hours to travel from a Swiss mountain village to another village.
Jane pedaled downhill at 20 km/h (kilometers per hour) and it took 2 hours to travel from a Swiss mountain village to another village.
If she pedaled back at 12 km/h how much time did it take?
 If a real estate agent received $60,000 as a 5% commission on the selling price of a house, how much was the house sold for?
If a real estate agent received $60,000 as a 5% commission on the selling price of a house, how much was the house sold for?
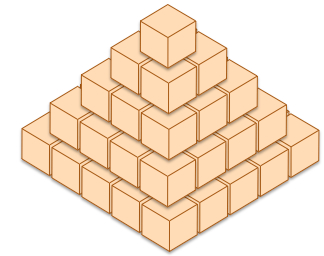 How much paint do you need to paint the pyramid if you need 1kg for 1 square surface?
How much paint do you need to paint the pyramid if you need 1kg for 1 square surface?
Don't count the bottom and the internal surfaces.
 A district sends, on average, a total of 200 million gallons of water each month to its 20,000 residential customers.
A district sends, on average, a total of 200 million gallons of water each month to its 20,000 residential customers.
In the winter months, it sends an average of 100 million gallons per month.
Assuming the winter period lasts three months, what is the average number of gallons sent in each nonwinter month?
 Gerry was born at 11:55 P.M. on December 31, 2000.
Gerry was born at 11:55 P.M. on December 31, 2000.
Jane was born 6 minutes later.
Which statement below is true?
 Anna (A), Bill (B), Cindy (C), and Daniel (D) work on a project.
Anna (A), Bill (B), Cindy (C), and Daniel (D) work on a project.
(1) Together, A, B, and C can complete it in 100 days.
(2) Together, B, C, and D can complete it in 101 days.
(3) Together, C, D, and A can complete it in 102 days.
(4) Together, D, A, and B can complete it in 103 days.
Rank them from the best to the worst performer.
 The picture shows a composite structure under stress.
The picture shows a composite structure under stress.
Estimate the percentage of strands that are broken.
 Computer Update Problem suggested by Leslie Green
Computer Update Problem suggested by Leslie Green
I spot a computer at work which is doing updates.
When I arrive it says '93% complete'.
I immediately start timing the activity and it takes 8 minutes to change to '95% complete'.
Assuming the 'percentage complete' relates directly to time, how much longer will it take to reach '100% complete'?
 A plastic ruler is much easier to bend in one direction than another according to the proportionality shown, where b is the breadth and d is the depth of the rectangular cross-section.
A plastic ruler is much easier to bend in one direction than another according to the proportionality shown, where b is the breadth and d is the depth of the rectangular cross-section.
The ruler can be approximated as being 1mm thick and 20mm wide.
How much stiffer is it in the hard-to-bend direction?
The problem was suggested by Leslie Green.
WARNING: Unless the ruler has some sort of anti-shatter statement written on it, do not try bending it like this without using eye protection. Older designs of rulers are known to shatter and eject bits of plastic into nearby eyes.
 Leslie Green asks:
Leslie Green asks:
Income tax is a strange concept when inflation is taken into account.
At the end of one year with a 1% interest rate and a 2% rate of inflation, what is the tax owed on a $10,000 saved amount, given a 20% income tax rate?
 Leslie Green, Oxfordshire, UK, asks:
Leslie Green, Oxfordshire, UK, asks:
"My neighbor a famous Professor of Physics has an excessively old and doddery gardener who knows absolutely nothing about basic science. Nevertheless this disrespectful old gardener insists that the Professor does not need to install an electric power cable all the way down to the ponds to power a fountain pump. The decrepit gardener insists that he has seen such a setup when he was a child and that it required no external power, had no electric motor, and could spray water well above the height of the source pond - but has no idea how. The Professor is still listening because the power cable will cost thousands of dollars to install.
What should he do?"
 Bob worked for 45 years. He did not work on weekends, had a 3-week summer vacation, and 7 days of official holidays. The hourly rate was $5 in the first year and it increased by $0.5 every year.
Bob worked for 45 years. He did not work on weekends, had a 3-week summer vacation, and 7 days of official holidays. The hourly rate was $5 in the first year and it increased by $0.5 every year.
He worked 8 hours per day.
What is his total income?
 There are two gods named Mendax and Fidelis, one on your left, the other on your right, but you do not know which is which. Fidelis always answers correctly. Mendax only answers one question in 7 correctly in a repeating cycle. You do not know which part of the cycle Mendax is currently on.
There are two gods named Mendax and Fidelis, one on your left, the other on your right, but you do not know which is which. Fidelis always answers correctly. Mendax only answers one question in 7 correctly in a repeating cycle. You do not know which part of the cycle Mendax is currently on.
You must determine which god is which using the minimum number of YES/NO questions. How many questions do you need to be certain?
To clarify the matter, if Mendax is answering incorrectly, your entire question is evaluated correctly and then the answer is reversed. A single question to both gods counts as two questions.
Author : Leslie Green
Inspired by G. Boolos. 'The hardest logic puzzle ever', The Harvard Review of Philosophy (6), 1996.
 An elephant weighing 2 tonnes (2 metric tons) and a rabbit weighing 1kg are balanced on a very long, perfectly rigid board.
An elephant weighing 2 tonnes (2 metric tons) and a rabbit weighing 1kg are balanced on a very long, perfectly rigid board.
If the elephant starts walking toward the center at the uniform rate of 1 meter per minute, how many km per hour must the rabbit run in order to maintain the balance?
Remember 1 tonne = 1000 kg
 If $1 equals 0.9 Euros, $1 equals 1.02 Swiss francs, and 1 euro equals 9.5 Norwegian kroner, who has the largest amount of money?
If $1 equals 0.9 Euros, $1 equals 1.02 Swiss francs, and 1 euro equals 9.5 Norwegian kroner, who has the largest amount of money?
 The ratio of women to men at a 100-employee company is 1 : 4.
The ratio of women to men at a 100-employee company is 1 : 4.
The company wants to change the proportion to 1 : 2.
How many men must be fired to reach the goal?
 Sasha is learning about Archimedes at school. His show-off sister is 3 years older than him and sets him a problem:
Sasha is learning about Archimedes at school. His show-off sister is 3 years older than him and sets him a problem:
A water butt is filled to the brim with water and then tilted to a 30° angle relative to the horizontal. A football has a lead weight sealed inside, but not fixed in position. The combined weight of the ball and weight is 330g. The ball floats to a depth of 1.2 inches.
Knowing the rough size of a football, estimate how much water spills out of the butt when the ball is lowered into the water gently.
Author : Leslie Green
 The largest denomination of currency ever printed by the Bureau of Engraving and Printing was the $100,000 Series 1934 Gold Certificate featuring the portrait of President Wilson. The notes were used only for official transactions between Federal Reserve Banks and were not circulated among the general public.
The largest denomination of currency ever printed by the Bureau of Engraving and Printing was the $100,000 Series 1934 Gold Certificate featuring the portrait of President Wilson. The notes were used only for official transactions between Federal Reserve Banks and were not circulated among the general public.
My friend Steve recently discovered this magic bill in his pocket as well as $16 in nickels and dimes.
If he exchanged all the money in his pocket for an equal number of $1, $2, $5, $10, $20, $50, and $100 bills, how many $100 bills did he receive?
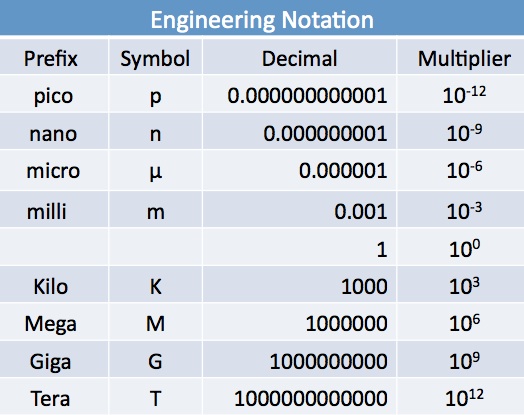 Leslie Green asks:
Leslie Green asks:
Engineers need to use quantities which can vary over at least 30 orders of magnitude. Using zeros such as 12300000000000 is impractical. Engineering notation uses a number between 1 and just less than 1000 followed by a standard multiplier for thousands, millions, and so forth.
Express the frequency of 3 thousand million Hertz (Hz) in engineering notation.
 The boss of a 10 person company is always the last to arrive, and gets the worst of the 10 car parking places as a result. Being the boss, he decides to allocate parking places to each of his 9 employees (all of whom use a car to get to work), obviously keeping the best parking place for himself.
The boss of a 10 person company is always the last to arrive, and gets the worst of the 10 car parking places as a result. Being the boss, he decides to allocate parking places to each of his 9 employees (all of whom use a car to get to work), obviously keeping the best parking place for himself.
The employees already hate the boss, who only got the job by marrying the owner. Further enraged by the new rule, they collectively decide to ignore it and just park randomly when they arrive. All places are good for the employees.
What is the chance that the boss gets to park in the best parking place?
Author: Leslie Green
 There’s a tennis tournament with one hundred players.
There’s a tennis tournament with one hundred players.
How many rounds does it take to determine a winner?
 One hundred workers complete a project in 100 working days.
One hundred workers complete a project in 100 working days.
Adding ten more people decreases the productivity of every worker by 1%.
How many days would it take to complete a similar project using 110 people?
 Gerry rents a red car from a Swiss car sharing company, which charges $3 per hour and $0.70 per kilometer (km).
Gerry rents a red car from a Swiss car sharing company, which charges $3 per hour and $0.70 per kilometer (km).
How far can he go with $80?
The speed limit is 120 km/h in Switzerland.
 Seven large pipes will drain a pond in six hours and thirty small pipes will drain the same pond in seven hours. How long will it take 3 large pipes and 6 small pipes to drain the pond?
Seven large pipes will drain a pond in six hours and thirty small pipes will drain the same pond in seven hours. How long will it take 3 large pipes and 6 small pipes to drain the pond?
 A pirate captain takes his pirate crew into the treasure cave shown. The Captain marks the sturdy plank in units of the depth of a pirate, meaning pirates can stand on the marked positions, but not any closer. All of the pirates, including the Captain, are the same weight, and the bag of Gold is one quarter the weight of a pirate.
A pirate captain takes his pirate crew into the treasure cave shown. The Captain marks the sturdy plank in units of the depth of a pirate, meaning pirates can stand on the marked positions, but not any closer. All of the pirates, including the Captain, are the same weight, and the bag of Gold is one quarter the weight of a pirate.
The Captain, being bold and fearless, is going to walk on the unsecured plank over the edge of the steep cliff to position 8 and collect the Gold. He does not worry about falling to his death in the bottomless pit below.
How many pirates are needed to stand on the plank to support the Captain?
Author: Leslie Green
 Alex bought a sports car for $48,000.
Alex bought a sports car for $48,000.
Two days later, he sold it to Betty for 5/8 the price he paid for it.
Three days later, Betty sold it to Craig for 1/6 less than she paid.
Four days later, Craig sold it to Alex for 4/5 what he paid.
Alex returned the car to the dealership and received his money back because it was a 10-day money back purchase.
How much money did Alex earn on the transactions?
 Cyclists are racing on a track. The first cyclist completed 200 km in 4 hours, and the second cyclist in 4 hours and 6 minutes.
Cyclists are racing on a track. The first cyclist completed 200 km in 4 hours, and the second cyclist in 4 hours and 6 minutes.
How much faster was the first cyclist?
 In a State, a vehicle licence plate always starts with 3 letters and ends with 3 digits, consisting of exactly 6 characters in total.
In a State, a vehicle licence plate always starts with 3 letters and ends with 3 digits, consisting of exactly 6 characters in total.
Estimate the largest possible number of different license plates in the State.
 Five rabbits bought a scale which is imprecise for small and large weights. It is OK for weights between 9 and 19 kg.
The rabbits weight themselves in pairs in every possible combination, resulting in the following:
Five rabbits bought a scale which is imprecise for small and large weights. It is OK for weights between 9 and 19 kg.
The rabbits weight themselves in pairs in every possible combination, resulting in the following:
10, 11, 12, 13, 14, 15, 15, 15, 15 and 16 kg.
How much does Benny Bunny weight if he is neither amongst the two heaviest nor amongst the two lightest rabbits?
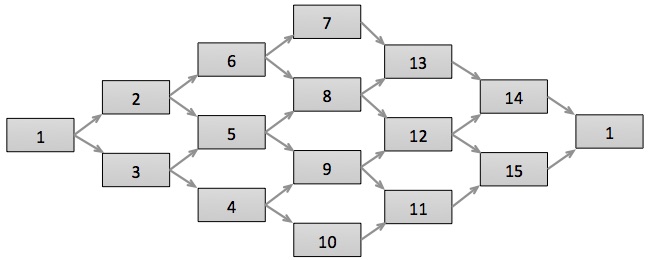 The picture shows a project plan. Arrows indicate where an activity must be finished before the next activity can start. The number shows the duration of the activity in days.
The picture shows a project plan. Arrows indicate where an activity must be finished before the next activity can start. The number shows the duration of the activity in days.
What is the duration of the project?
 Gerry’s electric bill was $125 for the month of July and $521 for the month of January. His heater was responsible for 20% of his electric bill in July.
Gerry’s electric bill was $125 for the month of July and $521 for the month of January. His heater was responsible for 20% of his electric bill in July.
If the rest of the bill is the same every month, how many dollars did Gerry spend on heating in January?
 Sixty percent of people living on the left side of the Long Street vote for the Left Party and forty percent don’t vote.
Forty percent of people living on right side vote for the Right Party and sixty percent don’t vote. There are sixty percent more voters on the right side than on the left side.
Sixty percent of people living on the left side of the Long Street vote for the Left Party and forty percent don’t vote.
Forty percent of people living on right side vote for the Right Party and sixty percent don’t vote. There are sixty percent more voters on the right side than on the left side.
If there are 780 voters on the Long Street, how many people voted for the Right Party?
 You need to buy several dozen chocolate Easter Eggs for a celebration. There are several vendors selling Eggs of the same quality in different-sized bundles.
You need to buy several dozen chocolate Easter Eggs for a celebration. There are several vendors selling Eggs of the same quality in different-sized bundles.
Which vendor is offering you the best deal per Egg?
 What is the fewest number of splittings I would need to break the bar into 24 small pieces?
What is the fewest number of splittings I would need to break the bar into 24 small pieces?
Each splitting consists of separating a single contiguous piece of chocolate along one of the grid lines.
 The black paint of the balls absorbs 95% of incident light, the remainder being reflected.
The black paint of the balls absorbs 95% of incident light, the remainder being reflected.
The white paint of the balls absorbs 5% of incident light, the remainder being reflected.
How many times are the black balls "blacker" than the white balls from the light absorption perspective?
 John's hourly wage increases from $18 to $19.5.
John's hourly wage increases from $18 to $19.5.
Estimate by how much his annual income increases.
Don’t take into account taxes.
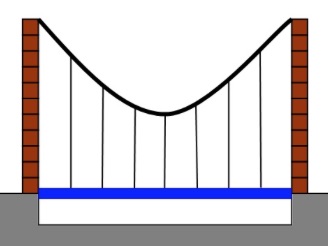 Johnny had a lesson at school which explained that ropes and cables are strong under tension but useless under compression. Brick and masonry, on the other hand, are strong under compression but weak under tension. Johnny has made the model suspension bridge (shown in the picture) using string and wooden blocks as a school project.
Johnny had a lesson at school which explained that ropes and cables are strong under tension but useless under compression. Brick and masonry, on the other hand, are strong under compression but weak under tension. Johnny has made the model suspension bridge (shown in the picture) using string and wooden blocks as a school project.
Comment on the design.
(Hint: consider the force on the top blocks)
Author: Leslie Green
 When only one small uniform solid cylinder of ice floats (with its axis vertical) in a glass of cold fresh water then 8.3% of the length of the cylinder sticks out of the water, the rest being submerged.
When only one small uniform solid cylinder of ice floats (with its axis vertical) in a glass of cold fresh water then 8.3% of the length of the cylinder sticks out of the water, the rest being submerged.
Estimate the specific gravity of ice.
(Reminder: Specific gravity is the ratio of the density of something relative to the density of cold pure water.)
HINT: Archimedes.
Author of the problem: Leslie Green
 The teacher likes to reward students for being smart. This year the top three students each get to pick 10 cacao beans from a large bag. Each student is blindfolded and wears a glove to do the selection. Beans are removed one at a time, inspected, then replaced, with the bag contents being thoroughly mixed before the next pick.
The teacher likes to reward students for being smart. This year the top three students each get to pick 10 cacao beans from a large bag. Each student is blindfolded and wears a glove to do the selection. Beans are removed one at a time, inspected, then replaced, with the bag contents being thoroughly mixed before the next pick.
The bag contains hundreds of fresh beans, and an equal number of already dried beans. The student who gets the most dried beans wins a big prize.
How does the best student optimise his chance of winning the prize?
Author: Leslie Green
 You are in a blue car on the side of the road. There is a lot of fast moving traffic going your way and you need to judge when to pull out into the traffic flow. If you want to wait for a really big gap in the traffic you could be stuck there for hours, so you need to pick a safe gap, but not an ideal huge gap.
You are in a blue car on the side of the road. There is a lot of fast moving traffic going your way and you need to judge when to pull out into the traffic flow. If you want to wait for a really big gap in the traffic you could be stuck there for hours, so you need to pick a safe gap, but not an ideal huge gap.
The traffic is going at an estimated speed of 56 mph (25 m/s). You are confident that you can get your car to accelerate from stationary to 25 m/s in 10 seconds (with constant acceleration).
Measuring the gap between the rear of your car and the front of the car behind, what is the minimum gap that would not cause an accident (we assume that the other driver does not brake)?
Author: Leslie Green
 A 1 ton car is heading due North at 60 mph. A 2 ton truck is heading due East at 30mph. There is sheet ice all over the intersection and the truck cannot stop. The truck smashes into the side of the car and the pair forms one tangled mess of bent metal. (Fortunately the drivers were wearing seatbelts and the air bags did their job. Nobody was injured.)
A 1 ton car is heading due North at 60 mph. A 2 ton truck is heading due East at 30mph. There is sheet ice all over the intersection and the truck cannot stop. The truck smashes into the side of the car and the pair forms one tangled mess of bent metal. (Fortunately the drivers were wearing seatbelts and the air bags did their job. Nobody was injured.)
In which direction does the mangled mess travel?
(Hint: conserve momentum not energy).
Author: Leslie Green
 An unknown chemical is found to lose 21 % of its original mass over a period of 20 years.
An unknown chemical is found to lose 21 % of its original mass over a period of 20 years.
What is its half life?
Half-life is the time required for a quantity to reduce to half its initial value. The term is commonly used in nuclear physics to describe how quickly unstable atoms undergo, or how long stable atoms survive, radioactive decay.
 Last year, Gerry worked at a summer camp. For each day that he worked, he earned $101 and he was not charged for food. For each day that he did not work, he was not paid and he was charged $19 for food. He spent on personal needs $5 everyday on average. After 21 working days, he had $696 in his pocket.
Last year, Gerry worked at a summer camp. For each day that he worked, he earned $101 and he was not charged for food. For each day that he did not work, he was not paid and he was charged $19 for food. He spent on personal needs $5 everyday on average. After 21 working days, he had $696 in his pocket.
How many days did he work?
 Alex (Adelaide, Australia), Gerda (Gelsenkirchen, Germany) and Craig (Calgary, Canada) want to talk using Skype videoconference.
Alex (Adelaide, Australia), Gerda (Gelsenkirchen, Germany) and Craig (Calgary, Canada) want to talk using Skype videoconference.
Adelaide time is GMT +10:30; the local time is 10:30 ahead of the Greenwich Mean Time (GMT or the UK time).
Gelsenkirchen time is GMT +1:00.
Calgary time is GMT -7:00.
How much time between 9:00 and 23:00 local time do they have for a conversation among all three together?
 Gerry has $100 in cash and many credit cards. He travels from New York to Paris, then to Geneva, back to Paris, and to New York.
Gerry has $100 in cash and many credit cards. He travels from New York to Paris, then to Geneva, back to Paris, and to New York.
Every time he moves, he exchanges the cash into local currency. The banks always take 5% commission.
If he does not spend the money, how much cash does he have at the end of the trip?
 2016 was a leap year: there were 29 days in February 2016.
2016 was a leap year: there were 29 days in February 2016.
January 1st, 2017 was on Sunday.
When will January 1st be on Sunday again?
 My house consumes only solar electricity and wind-turbine-generated electricity.
My house consumes only solar electricity and wind-turbine-generated electricity.
If there is 25% more solar energy cost than wind-turbine energy cost in my $45 monthly bill, how much do I pay for the solar energy every month?
 Martin saves $100 in the first school year, $200 in the second school year, $300 in the third school year and so forth.
Martin saves $100 in the first school year, $200 in the second school year, $300 in the third school year and so forth.
The amount of money he saved in the last year was $1,200.
How much money did Martin save in all?
 When my car's tank is a quarter empty my car can drive 240km more than when it is a third full.
When my car's tank is a quarter empty my car can drive 240km more than when it is a third full.
How many kilometers can it drive when the tank is full?
 Kevin cleans rooms in a student hostel; he earns twice as much for cleaning a large room as he does for cleaning a small room. Yesterday he cleaned five large rooms and three small rooms. If, instead, he had cleaned three large rooms and five small rooms, he would have earned $20 less.
Kevin cleans rooms in a student hostel; he earns twice as much for cleaning a large room as he does for cleaning a small room. Yesterday he cleaned five large rooms and three small rooms. If, instead, he had cleaned three large rooms and five small rooms, he would have earned $20 less.
How much did he earn yesterday?
 In a fictitious far away country income tax is not charged on the first $10,000 of annual income. After that, tax is charged at a 20% rate on the amount beyond the $10,000 allowance. If income exceeds the allowance by more than $30,000, however, earnings beyond that amount are taxed at 40%.
In a fictitious far away country income tax is not charged on the first $10,000 of annual income. After that, tax is charged at a 20% rate on the amount beyond the $10,000 allowance. If income exceeds the allowance by more than $30,000, however, earnings beyond that amount are taxed at 40%.
Arthur earns $20,000 per year. Barry earns $50,000 per year. How much more income tax (as a ratio) does Barry pay?
Author : Leslie Green
 My empty piggy bank weighed 300 grams.
My empty piggy bank weighed 300 grams.
Last year my piggy bank with money weighed 800 grams.
This year it weighed 1 kg (1000 grams).
With what percentage did my money's weight increase in a year?
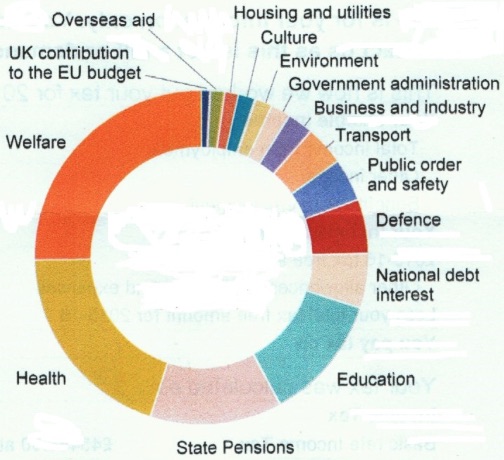 Leslie Green asks:
Leslie Green asks:
This is part of a real UK tax statement from 2015-2016 showing how the government spends Income Tax and National Insurance contributions.
Which statement is true?
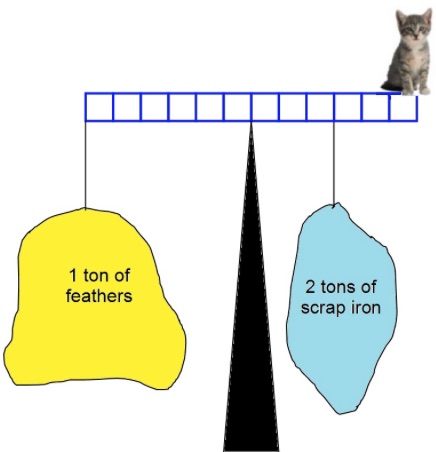 There is a real-life balance beam which is horizontal when unloaded. First we lock the beam in the horizontal position with a brake. Then we hang a 1 ton bag of feathers at a distance of 6 feet to the left of the pivot. Next we hang a 2 ton bag of scrap iron 3 feet to the right of the pivot. Charlie the cat is sitting about 6 feet to the right of the pivot.
There is a real-life balance beam which is horizontal when unloaded. First we lock the beam in the horizontal position with a brake. Then we hang a 1 ton bag of feathers at a distance of 6 feet to the left of the pivot. Next we hang a 2 ton bag of scrap iron 3 feet to the right of the pivot. Charlie the cat is sitting about 6 feet to the right of the pivot.
What happens when we release the brake?
Author: Leslie Green
 If it takes 5 men 5 days to dig 5 trenches in each of 5 countries, how long will it take 10 men to dig 10 trenches in one country?
If it takes 5 men 5 days to dig 5 trenches in each of 5 countries, how long will it take 10 men to dig 10 trenches in one country?
(We assume of course that the men have similar capacities to dig trenches, the countries make no difference to trench digging, and everything is just simple.)
Author: Leslie Green
 Leslie Green asks:
Leslie Green asks:
Can you guess why luxury cars are always long?
(Hint: consider bumps in the road)
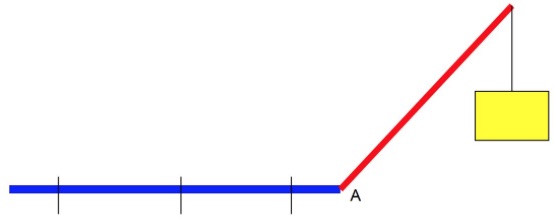 The heavy yellow weight is hung from the rigid red post. The blue plate is firmly attached to the ground with stakes (shown as thin vertical lines).
The heavy yellow weight is hung from the rigid red post. The blue plate is firmly attached to the ground with stakes (shown as thin vertical lines).
What can you say with certainty?
Author: Leslie Green
 The Ancient Olympic Games were held every four years.
The Ancient Olympic Games were held every four years.
They already existed at the time of Homer in 776 BC.
A Roman emperor banned the games in 393 AD.
If a Game was held in year 2 BC, which year of Common Era was the next Game?
The term anno Domini is Medieval Latin and often translated as in the year of our Lord. BC is before Christ. It should be noted that at the time of Homer they were not using the current Gregorian calendar. Historians have effectively relabelled the old dates to make them easier for us to understand. The Gregorian calendar is widely used, but it can be offensive to non-Christians to use the BC/AD terminology. Hence they are being replaced by BCE and CE, the CE meaning Common Era. Since this change only started happening around 2002, the older system is widely used and you should know both. - commented by Leslie Green
 Three spheres with equal diameters and equal masses are allowed to freely roll down a smooth inclined ramp.
Three spheres with equal diameters and equal masses are allowed to freely roll down a smooth inclined ramp.
The green sphere is homogeneous.
The blue sphere is hollow with a heavy rim.
The thin-walled orange sphere is hollow, with a smooth inner surface and filled with a dense, but remarkably inviscid liquid. (inviscid means having a very low viscosity - it's "runny")
When released at the same time, in which order do they arrive at the bottom of the ramp?
Author: Leslie Green
 A country has 20 towns. Each pair of towns is connected by a unique freeway (motorway). The freeway labels are consecutive positive integers beginning with 1.
A country has 20 towns. Each pair of towns is connected by a unique freeway (motorway). The freeway labels are consecutive positive integers beginning with 1.
What is the greatest number used to label a freeway in the country?
 Certain scientists think that love is a chemical state of mind and the formula for love is as follows:
Certain scientists think that love is a chemical state of mind and the formula for love is as follows:
C8H11NO2 + C10H12N2O + C43H66N12O12S2
dopamine + seratonin + oxytocin
A letter stands for a chemical element and the following number shows the number of atoms.
If the number is absent, then there is one atom.
How many atoms are there in the formula of love?
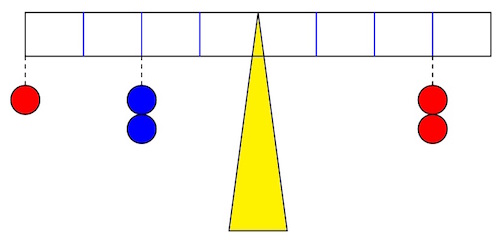 Leslie Green asks:
Leslie Green asks:
The beam is balanced by the spheres, all of which have the same diameter.
All of the red spheres have the same density as each other.
All of the blue spheres have the same density as each other.
What can you say with certainty?
 You have found the picture to the right on a random website on the internet. The author claims that it shows an inverted (upside down) red plastic funnel with nothing hidden inside. The author further claims that the top ring seems to almost float in the position shown, as if supported by springs.
You have found the picture to the right on a random website on the internet. The author claims that it shows an inverted (upside down) red plastic funnel with nothing hidden inside. The author further claims that the top ring seems to almost float in the position shown, as if supported by springs.
What could you reasonably conclude?
Author: Leslie Green
 Leslie Green asks:
Leslie Green asks:
Jane, a specialist in many different bird species, is trying to weigh a parrot. First she weighs a large glass bottle, then she weighs the same glass bottle with a parrot inside. The glass bottle is air-tight, so Jane makes sure that the parrot is never in the bottle for more than 3 minutes so it doesn't suffocate.
At first the bird stands on the bottom of the bottle so Jane takes a reading. Then the parrot hovers beautifully in the middle of the bottle for long enough that the weighing scale reaches a steady value. She records the result and releases the parrot.
Compare the two readings.
 There are 12 bills (notes) in my wallet.
There are 12 bills (notes) in my wallet.
If my girl-friend randomly takes out 10 bills from the wallet, she gets at least
one 1-dollar bill,
two 2-dollar bills, and
three 5-dollar bills.
How much money does she get at maximum?
 A fairly fit runner is in a race with other equally fit runners. The race distance is 1 mile. Unlike marathon runners, these runners do not take on fluids during the run.
A fairly fit runner is in a race with other equally fit runners. The race distance is 1 mile. Unlike marathon runners, these runners do not take on fluids during the run.
At the end of the run, what should we expect of our chosen athlete?
Author: Leslie Green
 Gerry drinks Swiss Mountain whole milk (3.9% fat), while Jane drinks fat-reduced milk (2.5% fat).
Gerry drinks Swiss Mountain whole milk (3.9% fat), while Jane drinks fat-reduced milk (2.5% fat).
If Jane drinks 1000 ml, how much does Gerry need to drink to get the same quantity of fat as Jane?
 Swiss car sharing system Mobility provides its 132,000 customers with 3,000 vehicles. They are usually red.
Swiss car sharing system Mobility provides its 132,000 customers with 3,000 vehicles. They are usually red.
On average, production of a new car generates emissions of CO2 of 15,000 kg = 15 tons.
If all these customers refuse to use the car-sharing system and buy a new car, how much additional CO2 is emitted into the atmosphere?
 Jane and Gerry put the marbles in a bag.
Jane and Gerry put the marbles in a bag.
Without looking, Jane takes a marble, then Gerry does.
What are the chances for him getting the red marble?
 Brian (aged 12), having been watching a Sci-Fi movie, is wondering what would stop a small airplane flying to the moon if we could somehow get it up into orbit.
Brian (aged 12), having been watching a Sci-Fi movie, is wondering what would stop a small airplane flying to the moon if we could somehow get it up into orbit.
Which of these do you think is the biggest problem?
A) There are no petrol stations on the way to the moon
B) Sound does not travel though space
C) The cabin is not air tight so the pilot couldn’t breathe.
D) The engine needs air to burn the fuel.
E) The engine needs air to cool down.
F) The wings need air to generate lift and control direction.
G) The propeller needs air to drive the plane forwards.
Author: Leslie Green
 A swimming pool has four taps.
A swimming pool has four taps.
The first can fill the pool with water in one day, the second - in two days, the third - in three days, and the fourth can fill the pool in four days.
Estimate how long it will take to fill the pool using all four taps together.
 Gerry, in his new red Ferrari, decides to make a 143-mile highway trip to Monterey to kiss his girl-friend Jane and immediately comes back by a two-lane ocean-side highway.
Gerry, in his new red Ferrari, decides to make a 143-mile highway trip to Monterey to kiss his girl-friend Jane and immediately comes back by a two-lane ocean-side highway.
Gerry starts the trip at 22:00 to avoid the traffic. His average speed equals the maximum speed for the road in question, which means he must have been breaking the speed limit!
The maximum speed limit on most California highways is 65 mph and 55 mph on a two-lane undivided highway.
If he is lucky enough to come back alive, at what time does he expect to be back?
 On most streets in the United States and Canada, odd numbers are on one side and even numbers on the other.
On most streets in the United States and Canada, odd numbers are on one side and even numbers on the other.
In many localities, addresses often increase by 100 for each cross street, so that the building numbers in each block vary only in the last two digits.
In Glory Street the number of houses is the same in each block, the first house number is 101 and the last is 2218.
How many addresses are there in Glory Street?
 A horse trough has a rectangular cross-section and is continuously being filled from a tap. Water is leaking from a hole in the bottom of the trough, the leak-rate being proportional to the square root of the water height in the trough.
A horse trough has a rectangular cross-section and is continuously being filled from a tap. Water is leaking from a hole in the bottom of the trough, the leak-rate being proportional to the square root of the water height in the trough.
The tap is adjusted so that the water rises to just under the top of the trough as a steady-state condition.
The tap is now adjusted to halve the flow rate.
What is the new steady-state height of water in the trough?
Author: Leslie Green
 An elite special forces soldier weighing 100 kg (with full kit) is being winched up to a steadily hovering helicopter at a constant speed of 5 m/s.
An elite special forces soldier weighing 100 kg (with full kit) is being winched up to a steadily hovering helicopter at a constant speed of 5 m/s.
What is the steady-state load on the winch cable?
NOTE: take the gravitational constant as 10 N/kg or 10 m/s2
Author: Leslie Green
 Two tiny motor boats set out from the shore of a lake, each with a full fuel tank and 4 full fuel cans. Each fuel can stores the same amount of fuel as held in the onboard tank. The two boats are tied together until it is time to separate them. The boats use fuel at a steady rate, regardless of the amount of fuel on board. One tank of fuel lasts for 30 minutes.
Two tiny motor boats set out from the shore of a lake, each with a full fuel tank and 4 full fuel cans. Each fuel can stores the same amount of fuel as held in the onboard tank. The two boats are tied together until it is time to separate them. The boats use fuel at a steady rate, regardless of the amount of fuel on board. One tank of fuel lasts for 30 minutes.
Each boat can only hold 4 full fuel cans, and a fuel can, once opened, has to be completely emptied into the fuel tank of the boat it is on. Fuel in the onboard tanks cannot be shared.
If fuel cans are optimally transferred, what is the maximum time one boat can continue on its journey?
Author: Leslie Green
 Three tiny motor boats set out from the shore of a lake, each with a full fuel tank and 3 full fuel cans. Each fuel can stores the same amount of fuel as held in the onboard tank. The three boats are tied together until it is time for one or more to separate. The boats use fuel at a steady rate, regardless of the amount of fuel on board. One tank of fuel lasts for 1 hour.
Three tiny motor boats set out from the shore of a lake, each with a full fuel tank and 3 full fuel cans. Each fuel can stores the same amount of fuel as held in the onboard tank. The three boats are tied together until it is time for one or more to separate. The boats use fuel at a steady rate, regardless of the amount of fuel on board. One tank of fuel lasts for 1 hour.
Each boat can only hold 3 full fuel cans, and a fuel can, once opened, has to be completely emptied into the fuel tank of the boat it is on. Fuel in the onboard tanks cannot be shared.
If fuel cans are optimally transferred, what is the maximum time one boat can continue on its journey?
Author: Leslie Green
 You are on a critical mission. You reach the shore of a lake where 55 similar tiny motor boats are moored. None of the boats have any fuel on board. There are 52 full cans of fuel available, with each can being the same capacity as the on-board fuel tanks of the boats.
You are on a critical mission. You reach the shore of a lake where 55 similar tiny motor boats are moored. None of the boats have any fuel on board. There are 52 full cans of fuel available, with each can being the same capacity as the on-board fuel tanks of the boats.
There are plenty of volunteers on hand to help you.
The boats use fuel at a steady rate, regardless of the amount of fuel on board. One tank of fuel lasts for 1 hour. Each boat can only hold 3 spare fuel cans, and a fuel can, once opened, has to be completely emptied into the fuel tank of the boat it is on. Fuel in the onboard tanks cannot be shared.
You need to get away as far as possible from this place by boat.
What is the maximum number of hours you can travel by boat?
Author: Leslie Green
 Leskie Green asks:
Leskie Green asks:
I have 4 books with weights of 1001g, 1003g, 1005g, and 1007g.
My balance scales will balance provided the nominal weights both sides are equal.
Using an optimum strategy, what is the minimum number of weighings which is guaranteed to find the heaviest book?
 Lenny throws a baseball to Kenny who is 20m away. The ball arrives in 500ms (0.5s).
Lenny throws a baseball to Kenny who is 20m away. The ball arrives in 500ms (0.5s).
Neglecting wind resistance and taking g = 10m/s2, to what height above the starting point does the ball reach, given that it is thrown from and received at the same height?
Although you may use different symbols, these formulae may provide some reminders:
s =ut + (1/2)at2 ; v2 - u2 = 2as ; v = u + at
Author: Leslie Green
 A table with four legs is resting on an uneven floor. Even if the floor were level, the table would still have been wobbly as the legs are not exactly equal.
A table with four legs is resting on an uneven floor. Even if the floor were level, the table would still have been wobbly as the legs are not exactly equal.
You have available a set of wedges to place under the legs to make the table secure. The table top is perfectly planar.
What is the minimum number of wedges required to make the table top secure and perfectly horizontal in the worst case of mismatched legs and floor?
Author: Leslie Green
 A particular electronic dictionary file of the English language contains 80,000 words, 1035 of which are three letter words.
A particular electronic dictionary file of the English language contains 80,000 words, 1035 of which are three letter words.
If a three letter word is chosen from random letters, estimate the chance that it will be a valid English word?
Author: Leslie Green
 Water is not getting from the PUMPING STATION to the FEED point. A section in the pipeline is blocked, and you are required to find the exact section which is blocked. You know that the pumping station is fine because you spoke to them on the phone.
Water is not getting from the PUMPING STATION to the FEED point. A section in the pipeline is blocked, and you are required to find the exact section which is blocked. You know that the pumping station is fine because you spoke to them on the phone.
You have been given an accurate sketched map of the pipeline showing 8 taps. If you open the tap and water comes out you know the pipe is unblocked up to that point. Using an optimum strategy, what is the minimum number of taps you have to open to establish which exact section of the pipe is blocked, assuming you are unlucky in your choices.
Author: Leslie Green
 Students, their parents, and teachers of Green-Planet school planted trees along a pathway. There are 120 trees in total. Every second tree is planted by students, and every third tree is either by teachers or by students.
Students, their parents, and teachers of Green-Planet school planted trees along a pathway. There are 120 trees in total. Every second tree is planted by students, and every third tree is either by teachers or by students.
The remaining trees are planted by parents.
How many trees did the teachers plant?
 Two ordinary dice lie on a table. The opposite faces of a die add up to 7.
Two ordinary dice lie on a table. The opposite faces of a die add up to 7.
What is the sum of the number of dots on all the faces that are visible from any side?
 In William Shakespeare's play "As You Like It ", Act 4, Scene 1, Page 3, Rosalind says
In William Shakespeare's play "As You Like It ", Act 4, Scene 1, Page 3, Rosalind says
"He that will divide a minute into a thousand parts . . . " (original text)
"A man who will dare to meet his lover even a thousandth part of a minute late . . . " (modern text)
Which of the following is equal to the distance the actor's sound goes for the mentioned time?
The speed of sound is 343 meter per second.
 Planets orbit around the Sun:
Planets orbit around the Sun:
Mercury 3 Earth months;
Venus 7 Earth months;
Earth 1 Earth year (365.25 days);
Mars 23 Earth months. Almost 2 Earth years;
Jupiter 142 Earth months. Almost 12 Earth years;
Saturn 354 Earth months. (29.5 Earth years);
Uranus 1009 Earth months. (84 Earth years);
Neptune 1979 Earth months (almost 165 Earth years);
Pluto 2977 Earth months (248 Earth years);
Gerry the Astronaut lived 25 years on the Earth, traveled 3 years to Mars, lived there for 12 Mars years, and traveled back to the Earth.
How old is he now?
 A claustrophobic gentleman chooses his place in the train that will enter a tunnel just after the station.
A claustrophobic gentleman chooses his place in the train that will enter a tunnel just after the station.
Where is the best place for him?
 Leslie Green asks:
Leslie Green asks:
The picture shows a fairly standard domestic refrigerator door.
We wish to consider the position of the tall glass bottle, neglecting any other items in the fridge.
The glass bottle is currently in the middle of the door. If we move it to the left it is closer to the door hinge. If we move it to the right it is closer to the open area.
Which is the best position for the tall glass bottle?
Hint: consider Newton.
 Every day over a period of 100 days three boys each sent a love letter to their unique and special girlfriends.
Every day over a period of 100 days three boys each sent a love letter to their unique and special girlfriends.
John always writes to Rosy, Pete always writes to Jade, and Steve always writes to Celeste.
The postman put the letters into three different boxes without looking at the name of the recipient.
What is the expected number of letters that reached the correct recipient?
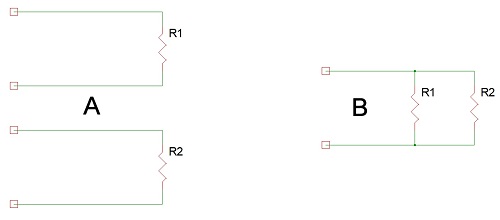 At present you have two heater elements supplied by two twin cables as shown in diagram A. The cables are old and damaged so you have decided to replace them with a single twin cable as shown in B. The system runs on low voltage DC so you feel safe to make the change. The heaters were permanently wired in parallel on the old system, so you have not made a change other than to the wiring.
At present you have two heater elements supplied by two twin cables as shown in diagram A. The cables are old and damaged so you have decided to replace them with a single twin cable as shown in B. The system runs on low voltage DC so you feel safe to make the change. The heaters were permanently wired in parallel on the old system, so you have not made a change other than to the wiring.
The old cables used to run quite hot so you make sure to double the cross-sectional area of the conductor in the new cable.
Have you made a satisfactory change?
Author: Leslie Green
 The image shows the cross-section of a simplified electrical water heater. The insulation is so good that we shall consider it to be perfect.
The image shows the cross-section of a simplified electrical water heater. The insulation is so good that we shall consider it to be perfect.
The design is based on an existing water heater which works well. However, management have decreed that the old heater is too big. Without changing the internal pipe structure significantly, the length of the heated section has been reduced by a factor of 10.
The water flow rate and electrical input power (2kW) have been kept the same. The characteristics of the insulation and heater element are unchanged compared to the previous design.
What is the most likely outcome?
Author: Leslie Green
 A computer programmer has characterised his new brick-built house methodically over a period of several years. He knows exactly how much heat to apply to the inside of the house for each and every condition of outside temperature, wind, rain, and humidity, when these are constant for a reasonable period of time (equilibrium conditions).
A computer programmer has characterised his new brick-built house methodically over a period of several years. He knows exactly how much heat to apply to the inside of the house for each and every condition of outside temperature, wind, rain, and humidity, when these are constant for a reasonable period of time (equilibrium conditions).
What happens when he runs his new temperature stabilisation program (assuming the program works as intended, in other words bug-free)?
by Leslie Green
 Leslie Green asks:
Leslie Green asks:
Kevin lives in a remote location and he often experiences power outages during poor weather conditions. He has resolved to build a battery backed-up solar and wind powered electrical system to maintain his power in any conditions. In order to design such a system he has measured his power usage over a typical winter's day.
Which are the essential readings from
1) Average power
2) Mean power
3) Minimum power
4) Maximum power
5) Standard deviation of power
6) Variance of power
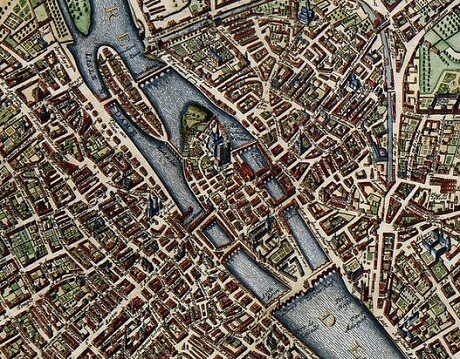 A newly married couple started walking and finished at the same point of Paris.
A newly married couple started walking and finished at the same point of Paris.
Was it possible to walk and cross over each of the bridges only once in 1657?
 The householder has realised that in due course the rug-rats will spontaneously evolve into large adults, and therefore the household hot water requirements will double. In order to double the hot water storage capacity the householder can either put in an extra identical hot water storage tank, or just put in a single double-capacity tank.
The householder has realised that in due course the rug-rats will spontaneously evolve into large adults, and therefore the household hot water requirements will double. In order to double the hot water storage capacity the householder can either put in an extra identical hot water storage tank, or just put in a single double-capacity tank.
Neglecting everything other than the heat-loss through the insulation, which solution gives a lower cost?
We assume that the hot water storage tank is just scaled up for the double-capacity tank, although the insulation thickness remains the same.
by Leslie Green
 Gerry bought a new car for $125,000 on January 1st 2015.
Gerry bought a new car for $125,000 on January 1st 2015.
The new car lost 20 percent of its value in its first year of ownership.
It is depreciated by 10% each following year.
What is the value of his car on January 2018?
 Leslie Green asks:
Leslie Green asks:
The photo shows a dial gauge caliper. (It has been edited to remove the manufacturer's name.)
What is the reading?
 Leslie Green asks:
Leslie Green asks:
The weather forecast shows an expected temperature of 5°C, but with the expected 10°C wind-chill, the weather map shows -5°C.
Assuming the weather forecast is spot on, will shallow puddles of water freeze?
[Remember that water freezes at 0°C.]
 In your experiment, you have been recording time in seconds from an electronic counting device.
In your experiment, you have been recording time in seconds from an electronic counting device.
You need to convert the reading of 5231 seconds into a normal time difference.
Use a calculator if you can.
 A top-fuel dragster, burning nitromethane rather than petrol (gasoline), is able to reach a top speed of 336 mph (150 m/s) in around 5 seconds.
A top-fuel dragster, burning nitromethane rather than petrol (gasoline), is able to reach a top speed of 336 mph (150 m/s) in around 5 seconds.
If we suppose that it accelerates at a constant value, what is that constant value?
by Leslie Green
 Leslie Green asks:
Leslie Green asks:
It is a sad fact of history that as technology evolves it can get harder for a student to get started with a new subject.
There is a lot of new terminology to learn which did not exist earlier on.
Books from 1940 (and earlier) talk about cycles per second. This is a fairly self-evident unit.
But what is the modern equivalent?
 30 discs, all of the same size, are placed into an opaque bag. 10 are made of aluminium, 5 are brass, 5 are wooden, 6 are made of ceramic, and 4 are magnets. The bag is shaken to mix all the discs up.
30 discs, all of the same size, are placed into an opaque bag. 10 are made of aluminium, 5 are brass, 5 are wooden, 6 are made of ceramic, and 4 are magnets. The bag is shaken to mix all the discs up.
A blindfolded person has to remove a disc from the bag using only a magnet on a string.
(If more than one disc is attached, a second person selects which disc is the closest to a particular mark on the magnet on the string, and only this disc is removed.)
What is the chance that the disc removed is a magnet?
by Leslie Green
 A toy car is released from stationary on a long smooth planar slope which is angled down relative to the horizontal.
A toy car is released from stationary on a long smooth planar slope which is angled down relative to the horizontal.
Describe the motion of the car (which was pointing directly downhill when released), assuming the wheel bearings have friction and that wind resistance is not negligible.
by Leslie Green
 Jim is calculating his income tax for the year. He earned $54,400. The document said that for incomes between $50,000 and $80,000, the tax is $10,000 plus 25% of any amount over $50,000.
Jim is calculating his income tax for the year. He earned $54,400. The document said that for incomes between $50,000 and $80,000, the tax is $10,000 plus 25% of any amount over $50,000.
How much tax will Jim owe?
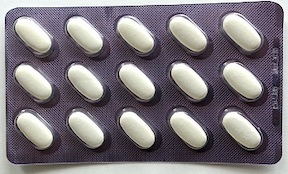 A certain medicament has a half-life of three hours. This means that every
three hours, half of the amount of the medicine is available in the bloodstream. The medicament is effective only when more than 50 mg is in the bloodstream.
A certain medicament has a half-life of three hours. This means that every
three hours, half of the amount of the medicine is available in the bloodstream. The medicament is effective only when more than 50 mg is in the bloodstream.
A patient receives 800 mg of the medicament at 11:00 am.
When should the next dose be given?
 Leslie Green asks:
Leslie Green asks:
There are three road vehicles, each having the same mass.
Which has the most powerful engine?